Maryam's Claim

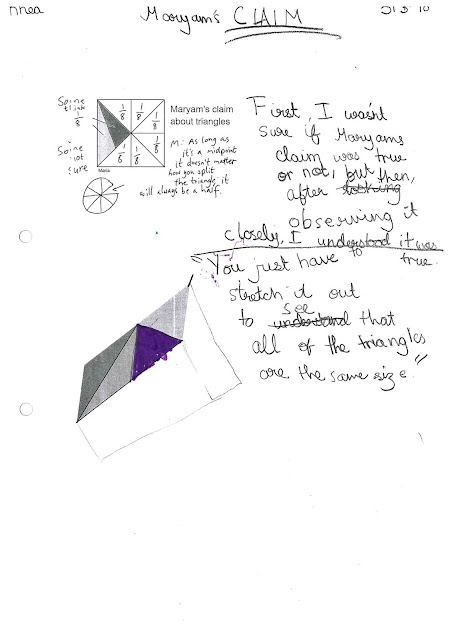
In 4G we've been looking at some squares we divided up, asking what fraction each part is. It's easy to see the eighths in this one, but what about the triangles on the left?

Then Maryam made her claim: "As long as it's the midpoint, it doesn't matter how you split the triangle; it will always be half." It was a really interesting mathematical claim, and one we had to really think about - Mr Gregg too!

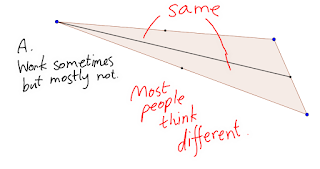
Most people didn't agree - the triangles on the left do not look like they have the same area.

We tried it on Geogebra. Still most people thought the two triangles had different areas.

John Golden suggested on Twitter that we see how you can get same-area triangles (if you start with the same "base"):

>


We also had a look at this:

This convinced a lot of us that Maryam's claim was true.

But Miguel was still not convinced:
"Technology isn't perfect. Is that really a proof?"

Dan Finkel suggested on Twitter that we change our triangle into a parallelogram and it will make it easier to see the truth:


We had a really good discussion about this, and quite a few people tried to persuade Miguel. Well done Miguel for getting us really thinking! There were all sorts of really good arguments to show how the two parts of the triangle in fact have equal areas.

After this we wrote down what we thought about it all: